Draw an angle in standard position calculatorterms of use – Draw an angle in standard position calculator terms of use are crucial for accurately representing angles in mathematics and related fields. This guide explores the process of drawing angles using calculators, highlighting their limitations and emphasizing the ethical considerations surrounding their use. We will delve into the definition of angles in standard position, exploring their components and visual representation.
Understanding these principles is vital for various applications, from basic geometry to complex engineering projects.
We’ll cover the practical steps involved in using different types of calculators to determine angle positions, including the necessary functions and step-by-step instructions. The guide will also demonstrate how to draw angles manually using a protractor and straightedge, and provide examples of real-world applications where this knowledge is essential. Finally, we will address potential misuse of tools and discuss responsible usage practices to ensure accurate and ethical application of these techniques.
Understanding Angles in Standard Position
Angles in standard position are fundamental in trigonometry and various fields of science and engineering. Understanding their components and representation is crucial for solving numerous problems. This section defines angles in standard position, details their components, and provides examples to clarify their representation.
Definition and Components of Angles in Standard Position
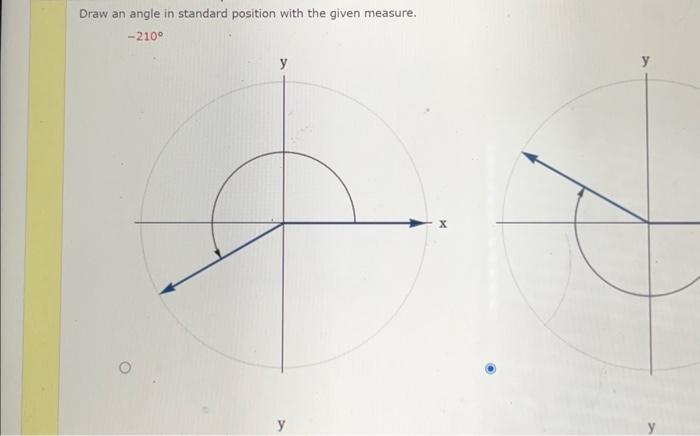
An angle in standard position is an angle drawn on a coordinate plane with its vertex at the origin (0,0) and its initial side lying along the positive x-axis. The terminal side is the ray that rotates from the initial side to form the angle. The amount of rotation determines the angle’s measure. Positive angles are measured counterclockwise from the initial side, while negative angles are measured clockwise.
Examples of Angles in Standard Position
Several examples illustrate the concept. A 45° angle has its terminal side in the first quadrant, while a 135° angle lies in the second quadrant. A -45° angle would be in the fourth quadrant. A 180° angle lies along the negative x-axis and a 270° angle lies along the negative y-axis.
Table Illustrating Angles and Their Positions
| Angle Measure (Degrees) | Angle Measure (Radians) | Initial Side | Terminal Side | Quadrant |
|---|---|---|---|---|
| 0° | 0 | Positive x-axis | Positive x-axis | None |
| 45° | π/4 | Positive x-axis | Between positive x and y axes | I |
| 90° | π/2 | Positive x-axis | Positive y-axis | None |
| 135° | 3π/4 | Positive x-axis | Between negative x and positive y axes | II |
| 180° | π | Positive x-axis | Negative x-axis | None |
| -45° | -π/4 | Positive x-axis | Between positive x and negative y axes | IV |
Calculator Use in Drawing Angles: Draw An Angle In Standard Position Calculatorterms Of Use
While calculators are invaluable for determining trigonometric values, their direct use in visually drawing angles in standard position is limited. This section details the calculator’s role in angle determination and provides a step-by-step guide.
Limitations and Calculator Types
Calculators cannot directly draw angles. Their primary function is to calculate trigonometric values (sine, cosine, tangent) and perform degree/radian conversions. Both scientific and graphing calculators can be used for this purpose; graphing calculators offer the additional benefit of visualizing the angle within a coordinate system, though the visual representation is often limited in precision.
Required Calculator Functions
The essential functions are degree/radian conversion and trigonometric functions. Accurate conversion between degrees and radians is necessary for calculations. Trigonometric functions help determine the position of the terminal side relative to the axes.
Step-by-Step Guide for Determining Angle Position
- Convert the angle measure to radians if necessary.
- Determine the quadrant based on the angle’s value (0-90°: Quadrant I, 90-180°: Quadrant II, 180-270°: Quadrant III, 270-360°: Quadrant IV). Negative angles follow a clockwise rotation.
- Use trigonometric functions (sine, cosine, tangent) to find the coordinates of a point on the terminal side. For example, if the angle is θ, a point on the terminal side could be (cos θ, sin θ).
- Plot the point on a coordinate plane and draw a ray from the origin through the point. This ray represents the terminal side of the angle.
Visual Representation of Angles
Manually drawing angles provides a clearer understanding of their geometric properties. This section Artikels the steps for accurately constructing angles using common tools.
Drawing Angles Using a Protractor and Straightedge
- Draw the initial side along the positive x-axis using a straightedge.
- Place the protractor’s center at the origin (0,0).
- Align the protractor’s 0° mark with the initial side.
- Locate the angle’s measure on the protractor.
- Mark a point at the angle’s measure.
- Draw the terminal side from the origin through the marked point using a straightedge.
Drawing Angles Using a Unit Circle
- Draw a unit circle (a circle with radius 1) centered at the origin.
- Determine the angle’s radian measure.
- Starting at the point (1,0) on the positive x-axis, move counterclockwise (for positive angles) or clockwise (for negative angles) along the circumference of the circle by the angle’s radian measure.
- The point where you stop represents a point on the terminal side of the angle. Draw a ray from the origin through this point.
Detailed Description of an Image Depicting Angles
Imagine a coordinate plane with the x and y axes clearly labeled. Several angles are drawn in standard position. A 30° angle is shown in the first quadrant, its terminal side extending into that quadrant. A 150° angle is in the second quadrant, and a -60° angle in the fourth quadrant. The initial side of each angle is along the positive x-axis.
Each angle is clearly marked with its degree measure. The coordinate system helps visualize the relationship between the angle and the Cartesian plane.
Applications and Examples
Understanding angles in standard position has far-reaching applications. This section demonstrates the drawing of specific angles and highlights real-world applications.
Drawing Angles of Various Measures
Drawing angles such as 30°, 45°, 60°, 90°, 135°, 180°, 270°, and 360° involves following the steps Artikeld earlier. These angles are particularly important as they represent common trigonometric ratios.
Acute, Obtuse, and Reflex Angles
Acute angles (0° to 90°) lie in the first quadrant. Obtuse angles (90° to 180°) are in the second quadrant. Reflex angles (180° to 360°) extend into the third and fourth quadrants. The method of drawing remains consistent regardless of the angle type.
Real-World Applications
Understanding angles in standard position is critical in navigation (determining bearing and direction), engineering (designing structures and mechanisms), and physics (analyzing forces and motion). For instance, in navigation, a bearing of 45° indicates a direction 45° counterclockwise from north.
Table Comparing Angles in Different Quadrants
| Quadrant | Angle Range (Degrees) | Sine | Cosine | Tangent |
|---|---|---|---|---|
| I | 0° – 90° | Positive | Positive | Positive |
| II | 90° – 180° | Positive | Negative | Negative |
| III | 180° – 270° | Negative | Negative | Positive |
| IV | 270° – 360° | Negative | Positive | Negative |
Terms of Use Considerations
Responsible use of angle calculators and related tools is paramount. This section addresses potential misuse and ethical considerations.
Potential Misuse and Ethical Considerations
Source: cheggcdn.com
Misuse could involve using incorrect calculator settings (degrees vs. radians), leading to inaccurate results. Ethical considerations arise when using angle calculators in situations requiring honesty and integrity, such as academic assessments or professional engineering projects. Presenting fabricated or manipulated angle calculations is unethical and could have serious consequences.
Responsible Use and Examples, Draw an angle in standard position calculatorterms of use
Responsible use involves double-checking calculations, understanding the limitations of the tools, and using them only for their intended purpose. Always verify results using alternative methods when possible. In academic settings, proper attribution and referencing of any calculation tools used should be adhered to.
Consequences of Misuse
Consequences can range from incorrect solutions in academic work to potentially catastrophic errors in engineering and other professional fields where accurate angle calculations are essential. Inaccurate calculations in navigation, for example, could lead to misdirection and potential danger.
Last Word

Source: cheggcdn.com
Mastering the art of drawing angles in standard position, whether using a calculator or manual methods, is a fundamental skill with wide-ranging applications. This guide has provided a comprehensive overview, from understanding the basic concepts to navigating the ethical considerations of utilizing technological tools. By adhering to responsible practices and understanding the limitations of calculators, users can ensure accuracy and integrity in their work.
Understanding the terms of use for a draw an angle in standard position calculator is crucial for accurate results. However, navigating online resources can sometimes lead to unexpected detours, such as stumbling upon unrelated sites like craigslist rockford personals , highlighting the importance of focusing on the intended application. Always ensure you’re using the calculator according to its specified guidelines for reliable angle measurements.
The ability to visualize and accurately represent angles is essential for success in numerous fields, making this skill a valuable asset for students and professionals alike.
